Lines of Force and Earnshaw's
Theorem
The great 19th Century chemist/physicist Michael
Faraday, who was not mathematically inclined but believed firmly in
facts, conceived the pictorial idea of "lines of force" which point
in the direction that something is being pushed and tell, by their
spacing, how strong the force is. The force is proportional to the
number of lines of force that pass through a small planar surface of
fixed area perpendicular to the line of force.
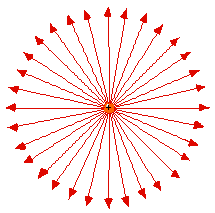
Electrical lines of force point in the
direction a positive charge would be pushed.
|
|
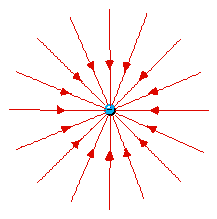
Lines of force surrounding an electron
(left) and a berylium nucleus (right). Naturally the force
(on a positive test charge) is toward the electron and away
from Be4+.
At first it seems strange that
the lines of force are only twice as close together for
the Be+4 as they are for the -1 electron. From
Coulomb's Law one would expect four times as large a
force. (click here for help on the idea of
Force Laws)
But these plots only show two of three
dimensions. The Be4+ lines are also twice as
dense in the direction perpendicular to the page. So
in 3D the lines of force are indeed four times as
dense.
|
|
This 2D vs. 3D geometrical argument shows
why lines of force work to describe an inverse-square force
law. The surface of a sphere surrounding a point charge increases in
area with the square of the radius, that is the distance from the
nucleus. But the same number of lines of force pass through this
sphere as pass through a smaller sphere closer to the charge. The
density of lines of force in 3D decreases with the square of the
distance, as it must do if they are to describe an inverse-square
force law.
|
To see whether you have
internalized this concept consider the following
problem:
What force
law could be expressed
by lines-of-force type diagrams in 2- (or 4-) dimensional
space?
|
Of course lines of force must originate on a
positive charge and teminate on a negative charge, which is
illustrated in the following complex diagram.
(No special effort was taken to make sure that the spacing
between lines of force is correct, so the diagram is only
qualitative):
(generated with
YP electric field shareware, which you can try for free if you
wish):
Consider this:
At a local energy minimum a positively
charged particle would have to feel a restoring force no matter which
way it is displaced. This could only be at a point where lines of
force all converge. Likewise, the only stable position for a negative
charge would be where lines of force all diverge. But each of these
conditions is true only for a point where there is a particle of the
OPPOSITE charge. By the same token, the only local energy
maximum for a particle is where there is another particle of the
same charge.
For a system governed by electrostatics there can
be no potential energy minimum, or maximum, in an unoccupied region
of space.
It is fairly easy to show mathematically that the
same is true for any combination of inverse square forces
(e.g. electrostatics plus gravity). This is Earnshaw's
Theorem, and it explains why you are not accustomed to seeing
things spontaneously levitating.
When Thompson proposed his plum-pudding
model of the atom, he nimbly sidestepped Earnshaw's Theorem by
putting the electrons inside a diffuse, positively
charged nucleus. Curiously, our present model of the atom is
precisely the opposite, with the point nucleus inside a
cloud of electron density. This is fine with Earnshaw, as far as the
location of the nucleus goes, but doesn't explain where the electron
cloud came from. That requires motion and the curious kinetic energy
of quantum mechanics.
copyright
© 2000 J.M.McBride